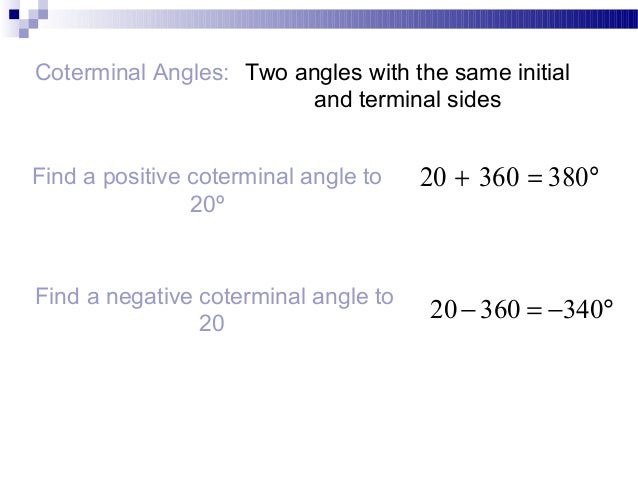
It is common called coterminal angle finder. Coterminal Angles are angles which share the same initial side and terminal sides. The side that defines the angle is called as the terminal side.
Finding coterminal angles is as simple as adding or subtracting 360° or 2π to each angle. There are an infinite number of coterminal angles that can be found.
Converting between degrees and radians can make working with angles easier in some applications.

For other applications, we may need another type of conversion.
When we study about lines and angles, we come across with different concepts related to angles. Colateral angles are one of them. This video demonstrates step by step how to find coterminal angles given an angle in degrees, theta. We do this by adding revolutions to are angle such as 3degrees or . Coterminal angles are angles that have the same initial and terminal sides as your original angle. How to find negative and postive coterminal angles in degrees and radians.
In the event that n=, then alpha and beta are coincident. Examples: a) Which of the following angles is coterminal with an . Tutoring video by Lone Star College-North Harris Math Department. If you are given an angle you can quickly find coterminal angles by adding or subtracti. Recall that when an angle is drawn in the standard position as above, only the terminal sides (BA, BD) varies, since the initial side (BC) remains fixed along the positive x-axis. If two angles are drawn, they are coterminal if both their terminal sides are in the same place - that is, they lie on top of each other.
For negative angles add 3instead). Sketch the angle to see which quadrant it is in. This video provides examples of determining coterminal angles in radian measure. We discuss how to find coterminal angles both in radians and i. Radian and Degree Measure - Duration: 26:42. The measure of an angle is determined by the amount of rotation from the initial side to the terminal side.
One way to measure angles is in radians. Arc length = radius when θ = radian .
No comments:
Post a Comment
Note: only a member of this blog may post a comment.